In Cambridge International’s Curriculum Programmes team, we are often asked how we design our curriculum and how we ensure progression of learning. Well, let me start by saying it is a complex, iterative and time-consuming process. It involves forwards, backwards, vertical and horizontal analysis of each learning objective, and for some subjects, this means we check a hundred or more learning objectives over and over again!
A helpful way to think about curriculum progression is as a logical sequencing of concepts, content and skills across multiple teaching stages, which helps learners develop more sophisticated ways of thinking. For Primary and Lower Secondary we look at progression from Stage 1 (age 5) to Stage 9 (age 14).
Forwards and backwards progression
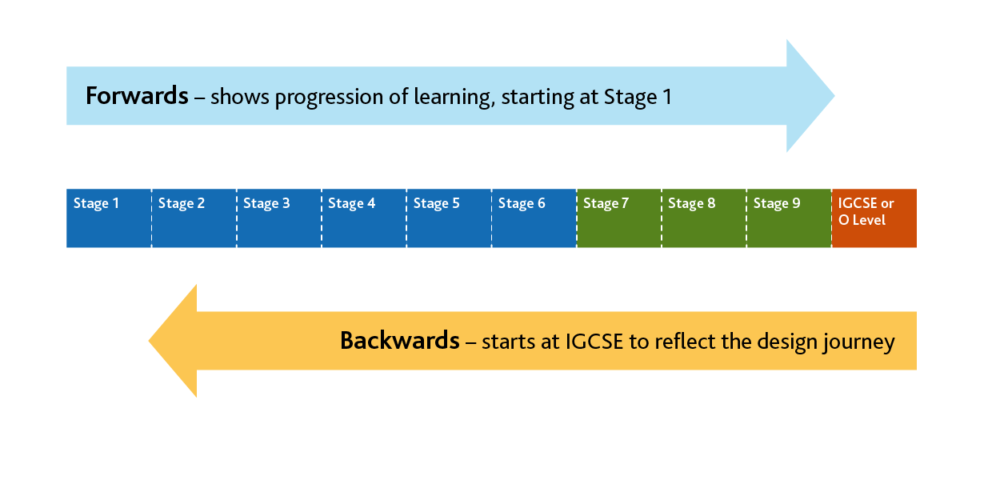
When we present the learning objectives in our Primary and Lower Secondary curricula, we show forwards progression, starting at Stage 1, then Stage 2 and so on as this is the order in which teachers and learners will experience our curriculum. However, when we design our Primary and Lower Secondary curricula, we actually start at Stage 9 and then consider what content, concepts and knowledge learners will need to understand at this level, to be prepared for IGCSE and O Level. Once it is clear what learners will need to know and do at Stage 9, we think what content to include in Stage 8 to enable learners to achieve Stage 9 and so on, all the way back to Stage 1. Throughout each stage we check to make sure everything is age-appropriate and that Stage 1 is a good starting point for the programme.
Although we use our progression model for all Cambridge Primary and Lower Secondary subjects, let’s take an example from our Cambridge Primary Mathematics curriculum to show each stage of the model. Our primary mathematics curriculum has three strands: Number; Geometry and Measure; and Statistics and Probability.
Backwards progression
Let’s start with backwards progression because that is the first step in our progression model. In this example, taken from our Mathematics Geometry and Measure strand I show how we first look at our IGCSE Mathematics and O Level syllabi to identify what learners need to be able to do so that they can carry out calculations involving the perimeter and areas of various 2D shapes. We then review international curricula and consult the latest mathematics research related to perimeter, area and 2D shapes, and consider what learners need to be able to do in Stage 9 to achieve these concepts. Then, we look at Stage 8 and so on backwards to Stage 1.
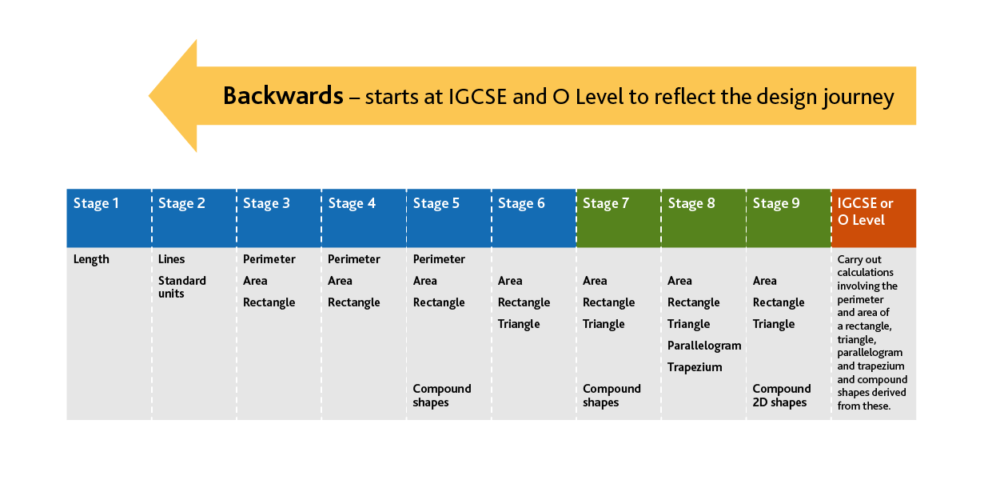
Forwards progression
Next, we look at forward progression. We look at each of the concepts (such as length, perimeter etc.) from Stage 1 to Stage 9 and write learning objectives that show progression of learning with an increasing level of demand. Using the same Geometry and Measure example, you can see how we show forwards progression of learning objectives in our curriculum frameworks starting at Stage 1. This explains to teachers and learners the order in which they first encounter the language of length in our curriculum before moving on to standard units, perimeter and area of a variety of 2D shapes so that they are able to calculate the perimeter and area of compound shapes at IGCSE or O Level.
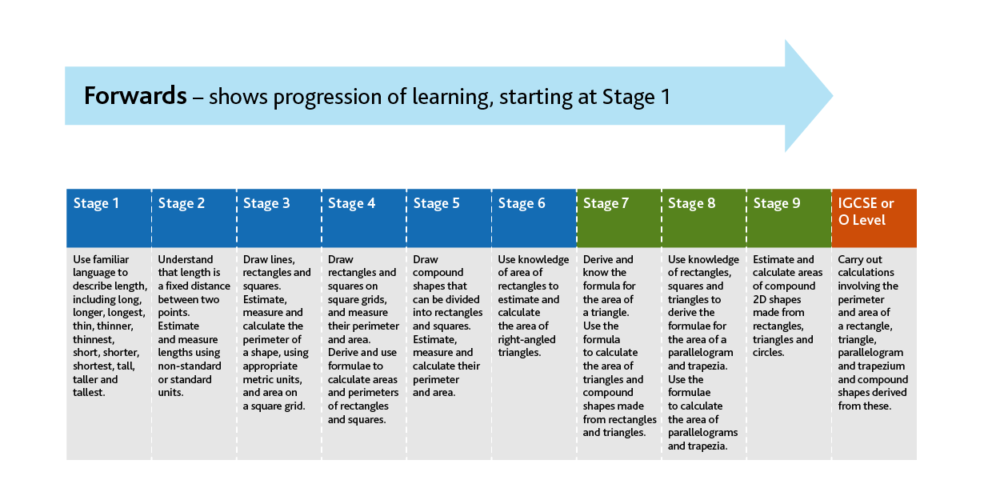
Progression up and down
In the Geometry and Measure strand, there are three sub-strands: Time, Geometrical reasoning, shapes and measurements, and Position and transformation.
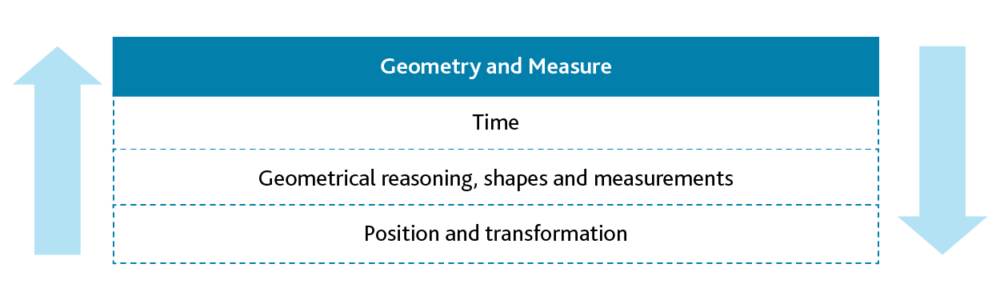
In this stage of development, we review the progression up and down each sub-strand to support learners in making connections between the concepts in different sub-strands. So, if they need to draw coordinates of 2D shapes in Stage 5 of the Position and transformation sub-strand, we make sure that they have learned about 2D shapes in an earlier stage so that they can now focus on learning about coordinates in the Geometrical reasoning, shapes and measurements sub-strand.
Progression across strands and subjects
In the next stage of our progression model, we check that there is alignment across each strand across each subject. So, for mathematics we review the learning objectives across Number, Geometry and Measure, and Statistics and Probability.

For example, in the Number strand we introduce decimals in Stage 5 so we make sure that decimals are not included in any learning objectives or assessments in Stage 4 or below in the other two mathematics strands, Geometry and Measure, and Statistics and Probability.
In the last part of our extensive progression model, we check alignment across subjects. We make sure that any mathematics concepts that are included in Science are introduced in Mathematics at least one stage earlier. For example, as decimals are introduced in Stage 5 Mathematics, learners will not be required to use decimals in Science until Stage 6. This allows learners to consolidate their knowledge of the concept in mathematics before they apply it to science.
Checking and rechecking alignment of learning objectives
Although I have described progression as a linear model in this blog, we actually use an iterative process. If we move even one learning objective in any direction, then it impacts all the other learning objectives. So, we have to continually check and recheck alignment forwards, backwards, up, down and across.
When you next read our curricula, you will know that we have been robust in our progression process so that you can focus on your teaching and your learners. If you are a registered Cambridge school, you can find progression grids for our Cambridge Primary and Lower Secondary subjects on our support sites https://primary.cambridgeinternational.org/ and https://lowersecondary.cambridgeinternational.org/
If you are not already registered with us but would like more information about our Primary and Lower Secondary programme, please enter your details here.